 |
| Looks science-y. What do you think his salary is? Intern, maybe? On an intern's salary, shaving is an expense that can be cut back on. |
From phyicsworld.com, here, it seems we're closing in on the electron's electric dipole moment, which, as we all know/forhot/never learned, should not be confused with its magnetic dipole moment.
Your daily dose of Wiki:
The electron electric dipole moment (EDM) de is an intrinsic property of an electron such that the potential energy is linearly related to the strength of the electric field: U=de·E. Within the standard model of elementary particle physics, such a dipole is predicted to be non-zero but very small, at most 10−38 e·cm,[1] where e stands for the elementary charge. The existence of a non-zero electron electric dipole moment would imply a violation of both parity invariance and time reversal invariance.[2] In the Standard Model, the electron EDM arises from the CP-violating components of the CKM matrix. The moment is very small because the CP violation involves quarks, not electrons directly, so it can only arise by quantum processes where virtual quarks are created, interact with the electron, and then are annihilated.[1] More precisely, a non-zero EDM does not arise until the level of four-loop Feynman diagrams and higher.[1] An additional, larger EDM (around 10−33 e·cm) is possible in the standard model if neutrinos are majorana particles.[1]
Experimentally, the electric dipole moment is too small to measure in all experiments to date. The Particle Data Group publishes its value as 0.07±0.07×10−26 e·cm. The most recent experiment performed at Imperial College London, placed an upper bound on (with a 90% confidence level) of |de| < 1.5×10−28 e·cm.[3]
Many extensions to the Standard Model have been proposed in the past two decades. These extensions generally predict larger values for the electron EDM. For instance, the various technicolor models predict dethat ranges from 10−27 to 10−29 e·cm.[citation needed] Supersymmetric models predict that |de| < 10−26 e·cm.[4] The present experimental limit is therefore close to eliminating some of these theories. Further improvements, or a positive result, would place further limits on which theory takes precedence.
References
- ^ a b c d Pospelov, M.; Ritz, A. (2005). "Electric dipole moments as probes of new physics". Annals of Physics 318: 119–169. doi:10.1016/j.aop.2005.04.002.
- ^ Khriplovich, I. B.; Lamoreaux, S. K. (1997). CP Violation Without Strangeness: Electric Dipole Moments of Particles, Atoms, and Molecules. Springer-Verlag.
- ^ Hudson, J. J.; Kara, D. M.; Smallman, I. J.; Sauer, B. E.; Tarbutt, M. R.; Hinds, E. A. (2011). "Improved measurement of the shape of the electron". Nature 473 (7348): 493–496. Bibcode 2011Natur.473..493H.doi:10.1038/nature10104.
- ^ Arnowitt, R.; Dutta, B.; Santoso, Y. (2001). "Supersymmetric phases, the electron electric dipole moment and the muon magnetic moment". Physical Review D 64: 113010. arXiv:hep-ph/0106089. Bibcode2001PhRvD..64k3010A. doi:10.1103/PhysRevD.64.113010.
and
In atomic physics, the electron magnetic dipole moment is the magnetic moment of an electron caused by its intrinsic property of spin.Contents
[hide][edit]Magnetic moment of an electron
The electron is a charged particle. Its angular momentum comes from two types of rotation: spin and orbital motion. From classical electrodynamics, a rotating electrically charged body creates a magnetic dipolewith magnetic poles of equal magnitude but opposite polarity. This analogy holds as an electron indeed behaves like a tiny bar magnet. One consequence is that an external magnetic field exerts a torque on the electron magnetic moment depending on its orientation with respect to the field.If the electron is visualized as a classical charged particle literally rotating about an axis with angular momentum , its magnetic dipole moment
, its magnetic dipole moment 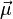 is given by:
is given by:
Here the charge is −e, where e is the elementary charge. The mass is the electron rest mass me. Note that the angular momentum in this equation may be the spin angular momentum, the orbital angular momentum, or the total angular momentum. It turns out the classical result is off by a proportional factor for the spin magnetic moment. As a result, the classical result is corrected by multiplying it with a correction factor.
in this equation may be the spin angular momentum, the orbital angular momentum, or the total angular momentum. It turns out the classical result is off by a proportional factor for the spin magnetic moment. As a result, the classical result is corrected by multiplying it with a correction factor.
The dimensionless correction factor g is known as the g-factor. Finally, it is customary to express the magnetic moment in terms of the Planck constant and the Bohr magneton:
[edit]Spin magnetic dipole moment
The spin magnetic moment is intrinsic for an electron.[1] It is:
Here is the electron spin angular momentum. The spin g-factor is approximately two:
is the electron spin angular momentum. The spin g-factor is approximately two:  . The magnetic moment of an electron is approximately twice what it should be in classical mechanics. The factor of two difference implies that the electron appears to be twice as effective in producing a magnetic moment as the corresponding classical charged body.The spin magnetic dipole moment is approximately one μB because
. The magnetic moment of an electron is approximately twice what it should be in classical mechanics. The factor of two difference implies that the electron appears to be twice as effective in producing a magnetic moment as the corresponding classical charged body.The spin magnetic dipole moment is approximately one μB because and the electron is a spin one-half particle:
and the electron is a spin one-half particle:  .
.
The z component of the electron magnetic moment is:
where mS is the spin quantum number. Note that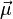 is a negative constant multiplied by the spin, so the magnetic moment is antiparallel to the spin angular momentum.The spin g-factor gs = 2 comes from the Dirac equation, a fundamental equation connecting the electron's spin with its electromagnetic properties. Reduction of the Dirac equation for an electron in a magnetic field to its non-relativistic limit yields the Schrödinger equation with a correction term which takes account of the interaction of the electron's intrinsic magnetic moment with the magnetic field giving the correct energy.For the electron spin, the most accurate value for the spin g-factor has been experimentally determined to have the value 2.00231930419922 ± (1.5 × 10−12).[2] Note that it is only two thousandths larger than the value from Dirac equation. The small correction is known as the anomalous magnetic dipole moment of the electron; it arises from the electron's interaction with virtual photons in quantum electrodynamics. In fact, one famous triumph of the Quantum Electrodynamics theory is the accurate prediction of the electron g-factor. The most accurate value for the electron magnetic moment is -928.476377 × 10−26 ± 0.000023 × 10−26J/T.[3]
is a negative constant multiplied by the spin, so the magnetic moment is antiparallel to the spin angular momentum.The spin g-factor gs = 2 comes from the Dirac equation, a fundamental equation connecting the electron's spin with its electromagnetic properties. Reduction of the Dirac equation for an electron in a magnetic field to its non-relativistic limit yields the Schrödinger equation with a correction term which takes account of the interaction of the electron's intrinsic magnetic moment with the magnetic field giving the correct energy.For the electron spin, the most accurate value for the spin g-factor has been experimentally determined to have the value 2.00231930419922 ± (1.5 × 10−12).[2] Note that it is only two thousandths larger than the value from Dirac equation. The small correction is known as the anomalous magnetic dipole moment of the electron; it arises from the electron's interaction with virtual photons in quantum electrodynamics. In fact, one famous triumph of the Quantum Electrodynamics theory is the accurate prediction of the electron g-factor. The most accurate value for the electron magnetic moment is -928.476377 × 10−26 ± 0.000023 × 10−26J/T.[3][edit]Orbital magnetic dipole moment
The revolution of an electron around an axis through another object, such as the nucleus, gives rise to the orbital magnetic dipole moment. Suppose that the angular momentum for the orbital motion is . Then the orbital magnetic dipole moment is:
. Then the orbital magnetic dipole moment is:
Here gL is the electron orbital g-factor. The value of gL is exactly equal to one, by a quantum-mechanical argument analogous to the derivation of the classical gyromagnetic ratio.[edit]Total magnetic dipole moment
The total magnetic dipole moment resulting from both spin and orbital angular momenta of an electron is related to the total angular momentum J = L+S by:
The g-factor gJ is known as the Landé g-factor, which can be related to gL and gS by quantum mechanics. See the article on Landé g-factor.[edit]Example: Hydrogen atom
For a hydrogen atom, an electron occupying the atomic orbital Ψn,l,m, the magnetic dipole moment is given by:
Here is the orbital angular momentum, n,l and m are the principal, azimuthal and magnetic quantum numbers. The z-component of the orbital magnetic dipole moment for an electron with a magnetic quantum number ml is given by:
is the orbital angular momentum, n,l and m are the principal, azimuthal and magnetic quantum numbers. The z-component of the orbital magnetic dipole moment for an electron with a magnetic quantum number ml is given by:
[edit]See also
- Anomalous magnetic dipole moment
- Nuclear magnetic moment
- Fine structure
- Hyperfine structure
- g-factor
[edit]Notes
- ^ A. Mahajan and A. Rangwala. Electricity and Magnetism, p. 419 (1989). Via Google Books.
- ^ http://physics.nist.gov/cgi-bin/cuu/Value?eqae%7Csearch_for=electron+magnetic+moment
- ^ http://physics.nist.gov/cgi-bin/cuu/Value?muem%7Csearch_for=magnetic+moment+electron


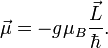
 is the
is the 



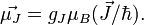


1 comment:
I made a post on EDM etc. earlier. Would be happy for some feedback, because I am no expert.
http://zone-reflex.blogspot.com/2011/02/edm-reveals-antimatter-without-mssm-and.html
Post a Comment